I have done a lot of research on the concept of the ideal solar panel installation angle. I even dedicated this site to it. Through various experiments, I came up with interesting and surprising results.
What is proper solar panel angle
The sun’s rays fall at various angles during the day. The ideal angle of inclination of the solar panel is the one that brings us the highest yield of solar energy that we convert into electricity. It is imperative that the angle of the incident light be at the right angle to the solar panel. This goal is impossible to achieve with fixed solar panels because the earth rotates around the sun and, therefore, the angle of incidence of light rays. The only way to keep the light falling at the right angle all day is to use a 2-axis tracking system. It is a complex electro-mechanical system that rotates the solar panel during the day so that it follows the position of the sun every second. We will discard this system for further discussion since most mini solar power plants on the roof or ground are fixed. What we are interested in is the angle at which we should place the solar panel in order to make the most of it. Or, in other words, the angle of incident light in relation to the surface of the solar panel should be as close as possible to 90 degrees. A general, unwritten rule used by many solar panel installers is to install the panels at an angle equal to the latitude of the installation site. You can find latitude on the Compass app on your mobile phone, on Google Maps, or on a regular map.
Proper solar panel angle = latitude ???
Some authors recommend the following formula:
Proper Solar Panel Angle = 0.9 * Latitude
I have tried both and concluded that they are not reliable formulas. The SPAC application uses, in some cases, a formula that is a stochastic factor and relies on climatic characteristics and monthly insolation. But since it can’t be 100% accurate either, it’s best to do a little experiment with the SPAC application and solve that dilemma.
How could I find the ideal angle and placement
In all parts of the northern hemisphere, solar panels should face south. Fixed solar panels should be mounted at an ideal angle. Rotating the panels around one axis can increase their efficiency. One case of turning the panel around its axis is the seasonal setting of the tilt of the solar panel. For example, in winter, the sun is “quite low,” so the favorable winter angle is as large as possible. In summer, when the sun is “high,” a smaller angle of inclination of the solar panel is advantageous. These are purely geometric dispositions and depend only on the day of the year. The SPAC app gives you “seasonal angles.” And you can apply it if you set up your mini solar power plant on the ground with a simple mechanism to change the angle of inclination, similar to reclining a beach chair. However, the profit from this setup barely reaches 7%. The second and most effective method of one-axis tracking is the rotation of the panel around an axis that is at the angle of inclination of the panel from morning to sunset. This system can reach maximum performance, almost like 2-axis tracking. If you have the opportunity, I recommend you use it. However, when solar panels are typically mounted on the roof, there is limited flexibility for adjustments. On the other hand, when installed on the ground, we have the freedom to strive to determine the optimal fixed angle. I will use the interpolation method until I get the maximum annual electricity income.
Using application SPAC for searching ideal angle
For one latitude, we cannot use the same formula because of the differences in the climate of various areas. In some places there are monsoons, and in others there are droughts; somewhere it is windy and there are no clouds; and somewhere there are a lot of fumes. Even the difference in temperature affects the operation of the solar panels, thus the determination of the ideal angle of inclination. I will divide the experiment into two groups. In the first one, I will consider the gain from solar energy in the cities located on the so-called “Tropic of cancer.” It is a meridian of geographic latitude 23° 26′ 22”. Its characteristic is that on June 21, the sun falls at a perpetual angle.
I will examine cities that are approximately at that latitude in Asia: Taichung in Taiwan and Muscat in Oman. In North Africa: Marsa Alam, Egypt, and Nouadhibou, Mauritania And in North America: Monterrey, Mexico, and New Orleans in the USA.
In the second case, I will examine the cities at a latitude of 45 degrees. In most cases, it is the beginning of an area with a temperate continental or continental climate. In North America, I will examine Seattle and Vancouver. In Europe, Monaco and Bucharest are the capitals of Romania; in Asia, Ulaanbaatar is the capital of Mongolia.
In all cases, I will adopt the following parameters: Orientation towards the south (0 degrees). You must enter zero! The tilt angle of the panel will be a series starting at latitude I and decreasing in steps of 2 or 3 degrees, for example [29, 27,…] until I get the maximum annual yield of electricity. An array of 10 Panasonic 400 W solar panels mounted on the ground. Must check “Ground”
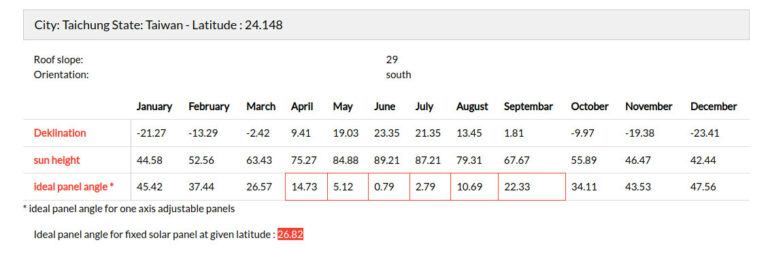
First table on the result page. We will check “Ideal panel angle for fixed…” for the given latitude. Step by step, all values in the array [29, 26, 23, 20]. For example, if we find the maximum between 26 and 23, we will check 25 and 24 degrees.
The calculation flow for all cases looks like this:
1. Entry of parameters.
Calculation and review of only the data on the annual profit, and it all looks like a table.
After that calculation, I will make table with degrees and anual production.
Results for cities on the approximate geodetic plane of 23.439 degrees (Tropic of Cancer)
Thaichung - Taiwan, Latitude: 21.48
| Panel slope | 29 | 26 | 26.8 | 23 | 20 |
|---|---|---|---|---|---|
| kWh/year | 4350.85 | 4351.69 | 4352.52 | 4342.33 | 4323.17 |
Muscat - Oman , Latitude 23.61
| Panel slope | 29 | 26 | 24.8 | 23 |
|---|---|---|---|---|
| kWh/year | 7459.87 | 7510.37 | 7510.96 | 7506.85 |
Marsa Alam – Egypt, Latitude: 25.067
| Panel slope | 29 | 26 | 24.9 | 23 | 22 | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| kWh/y | 8312.11 | 8339.99 | 8345.21 | 8349.82 | 8348.92 |
Nouadhibou – Mauritania, Latitude: 20.93
| Panel slope | 29 | 26 | 23 | 22 | 20 | 18 |
|---|---|---|---|---|---|---|
| kWh/y | 6730.35 | 6764.67 | 6781.08 | 6783.02 | 6782.01 | 6774.32 |
.jpg)
